ISSA Proceedings 1998 – What Makes The Reductio Ad Absurdum An Important Tool For Rationality?

This paper presents a summarized chapter from a study on the Reductio ad Absurdum, in which its logical, semantical and epistemological aspects are analyzed. I here focus on the neo-rationalistic motivation behind this research. The following analysis is only a partial report, in need of further study.
Traditional rationality is the quest for certainty and knowledge. It characterizes specific beliefs which are derived on the basis of appropriate reason and specific appropriate principles of assessment. The story of its failure is the story of the success of skepticism. One of the answers to the skeptical challenge on rationality is the conceptual shift from the notion of ‘verification’ to that of ‘refutation’. However, if refutation is understood as certainty regarding the falsity of the refuted, then this shift is only superficial, and does not solve the basic challenge. Certainty regarding a falsity is no less subject to the skeptical challenge than certainty regarding truth. My proposal to a solution to this problem is based on a modification to the common epistemological understanding of the Reductio ad Absurdum mode of argumentation. The key idea is to see refutation as conditional reasoning instead of absolute or certain, and to see rationality as focusing on the process of reasoning instead of its outcome.
The intense criticism on the notion of verification and the shift to that of refutation is best known through the work of Karl Popper. The paradigmatic examples of this shift, elaborated by Popper and his followers, pertain to science. The notion of refutation is, however, by far more problematic when it comes to philosophical controversies. There aren’t notions of crucial experiment and of fact of the matter in the non-empirical contexts of philosophical controversies, even in principle.
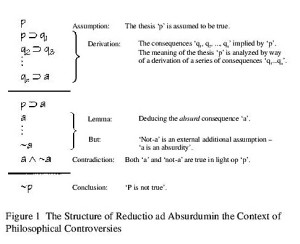
The Reductio ad Absurdum mode of argumentation is a basic logical tool in the procedure of refutation. The application of refutations to philosophical controversies must, therefore, account for the structure and function of the Reductio ad Absurdum. In a Reductio ad Absurdum, one starts by assuming the truth of a thesis ‘p’ (see first below). The meaning of the thesis ‘p’ is analyzed by way of deriving a series of consequences ‘q1’ to ‘qn’ implied by the assumed thesis. This clarification of the meaning of the thesis ‘p’ ends in the derivation of the consequence ‘a’. The consequence ‘a’ becomes an absurdity, however, in light of an external additional assumption regarding the truth of its negation ‘not-a’. The ensuing contradiction ‘a and not-a’ leads to the conclusion that the thesis ‘p’ is not true, namely that ‘not-p’.

I want to begin my suggestion with the following problem: From a logical point of view, every indirect argument scheme of inferring a conclusion from a given set of premises, such as the Reductio ad Absurdum, can be rephrased as a direct and constructive one. In what sense, then, is the Reductio ad Absurdum preferable to a direct proof that ‘not-p’ ? The Reductio ad Absurdum can be interpreted or understood in at least three ways, of which only one makes it preferable to a direct proof.

Figure 1 The Structure of Reductio ad Absurdumin the Context of Philosophical Controversies

The first is used in mathematics. In it, the absurd consequence ‘a’, implied by the thesis ‘p’, is supposed to be necessarily false. Furthermore, its negation ‘not-a’ is also implied by the thesis ‘p’, and thus internal to it. The ensuing contradiction ‘a’ and ‘not-a’ is, therefore, a conclusion of the thesis ‘p’. Consequently, the negation of the given thesis ‘not-p’ is deemed necessarily true. This kind of Reductio ad Absurdum must assume the Law of the Excluded Middle.
The second mode of Reductio ad Absurdum differs from the first with regard to the nature of the absurd consequence ‘a’. In this mode, the absurd consequence is not necessarily false, but its falseness or improbability can be established to at least some degree of certainty. The variety of possible justifications for rejecting the absurd ‘a’, or accepting ‘not-a as true, will not be discussed here; for the present purpose, any theory of statement evaluation will do. This mode of Reductio ad Absurdum, like the first one, assumes the Law of the Excluded Middle on the way to proving the truth of the thesis ‘not-p’. The degree of certainty regarding the truthfulness of the thesis ‘not-p’ is based on the degree of certainty attached to the falseness of the absurd consequence ‘a’. This mode of argumentation is also known in the Stoic logic as Reductio ad Impossibile. There are two crucial presuppositions common to the mathematical usage of Reductio ad Absurdum and to its weakened version as Reductio ad Impossibile: one is the ability to establish the falseness of the absurd consequence ‘a’; the second is the Law of the Excluded Middle. These two presuppositions are susceptible to skeptical criticism, regarding the vulnerability of any knowledge claim, and regarding the heavy metaphysical and epistemological background attached to the Law of the Excluded Middle.
The third mode of Reductio ad Absurdum is a further weakening, since it does not necessarily satisfy these two presuppositions. The reason is that this mode of Reductio ad Absurdum deals with philosophical theses. First, the negation of the absurd consequence ‘not-a’ is not necessarily a consequence of the thesis ‘p’, nor can its truthfulness be established with certainty. Second, the Law of the Excluded Middle is not presupposed. The falseness attributed to the absurd consequence ‘a’ shows nothing but that the thesis ‘p’ cannot hold. Without the metaphysical and epistemological background of the Law of the Excluded Middle, the proved proposition is, therefore, ‘p is disproved’. This mode can disprove a given thesis ‘p’ but cannot prove the truth of its negation ‘not-p’. The false or absurd consequence ‘a’ of the thesis ‘p’ shows that ‘p’ cannot hold and the conclusion that ‘not-p’ expresses just that. This logical characteristic makes the Reductio ad Absurdum fundamental to the possibility of rational reasoning, since various logical and metaphysical criticisms on classical logic and its presuppositions, such as Intuitionism, do not hold in this case. In the following I will concentrate only on this weakened version of the Reductio argument.

Given the above logical distinction, it is clear that the stage in which the absurd consequence a is negated, is a crucial element in the logical operation of the Reductio ad Absurdum. This negation leads to the contradiction ‘a’ and ‘not-a’, whereby the thesis ‘p’ is disproved. ‘Not-a’ is, however, an additional assumption, external to the thesis ‘p’, that can come either from the same theory, to which ‘p’ belongs, or from some other theses or facts.
The epistemic meaning of negating the absurd consequence ‘a’ is crucial to the understanding of the Reductio ad Absurdum as a rationalistic tool. What is the meaning of the negation operation in general? It is not, to be sure, its meta-linguistic truth table. The truth-table is only the schema for performing a negation with regard to a specific statement. But what does ‘not-a’ mean? The clue is that the sense of ‘not-a’ is the semantical and epistemological complement of the sense of ‘a’.
The epistemic aspect of the use of the Reductio ad Absurdum is the conviction that either the absurd ‘a’ or its negation ‘not-a’ is false, namely, that they are complementary. Since we are not assuming the Law of the Excluded Middle, ‘a’ and ‘not-a’ can both be false, though can not both be true. This is part of the more general conviction not to accept contradictions, which is itself a matter of philosophical and epistemological dispute. Contradictions induce the changing of philosophical theories only if this conviction is given. This conviction is not trivial nor necessary. But adopting it is essential if we insist upon rational grounds for changing theories.

In what sense is the Reductio ad Absurdum rational? The core of traditional rationality is the quest for a specific sort of certainty, an humanistic certainty as opposed to a divine one. This trend is subject to the skeptical criticism on the possibility of demonstrating infallible propositions. According to my suggestion, there are some characteristics of Reductio ad Absurdum which are definitely rationalist:
First, Reductio ad Absurdum arguments point to the unacceptability of theses rather than the truth of their negation. In principle, every philosophical thesis is debatable and there are no clear cut proofs or disproofs. But the rationalist intent requires that there be a way to elucidate the controversies in a way that will eventually lead to eliminating unacceptable theories by way of refutation, even if this refutation is only conditional and not absolute. The notion of intellectual progress, so important to traditional rationality, is, therefore, retained in the weakened form of conditional refutation and a proof up-to-a-point.
Second, the use of Reductio ad Absurdum circumscribes the skeptic criticism of deduction as a tool for attaining new knowledge. By bringing in the ‘external’ assumption that the negation of the absurd ‘not-a’ is true, in order to evaluate the thesis ‘p’, the Reductio argument makes us aware of connections between remote areas of knowledge, hitherto hidden. Since ‘not-a’ was previously deemed irrelevant or external to the theory to which the thesis ‘p’ belongs, evaluating ‘p’ in light of ‘not-a’ amounts to a kind of new knowledge. This way, the use of Reductio ad Absurdum reestablishes the traditional rationalist role of logic in clarifying disputes and attaining new knowledge.
Third, in eliminating the more implausible theses, the Reductio ad Absurdum retains a weakened form of the distinction between the correct and the incorrect. Rationality does not necessarily assume that any dispute must end in isolating all and only the true and evident theses. It does, however, say that there is a crucial difference between acceptable and unacceptable theses. The Reductio ad Absurdum opens a way to circumscribe the skeptical obstacle and retain a core of traditional rationality.

This analysis of Reductio ad Absurdum equates rationality with the use of logic as a tool for criticism. No better certainty can be reestablished in light of skepticism than a conditional one. The Reductio ad Absurdum does not reestablish rational certainty, but offers a last resort in the form of conditional certainty. It can be seen as a partial answer to skepticism, that preserves the substance of rationality.
It is ironic that the Reductio ad Absurdum mode of argumentation joins forces with an important trend in skepticism. Using the paradox of entailment, namely that contradictions entail any statement, the Reductio argument forces opponents to admit contradictions, and to abandon their stands or amend them. That way, Reductio ad Absurdum and skepticism both discuss philosophical theses with the aim of eliminating the more implausible and dubious ones. The coincident use of Reductio ad Absurdum and skepticism lasts, however, only as long as the goal is to block the way to nihilistic conclusions implied by epistemological skepticism.

Traditional rationalist philosophy states that there are justified knowledge claims of a specific sort, mainly in formal logic, mathematics and science. These specific statements must succumb to skeptical criticism. If anything of this tradition is to be retained, it must undergo a profound change. The change suggested here is the identification of rationality with the process of logical disproof instead of identifying it with some set of knowledge claims. The weight is transferred from the proved statements to the process of disproving.
The shift in the essence of rationality is best exemplified with regard to the question of choosing a logical system. Traditional rationality is identified with classical logic, and would, therefore, break down in light of the different and not-equivalent systems of logic. The change suggested here alters the status that classical logic enjoys in traditional rationality and thus circumscribes this fatal obstacle. Instead of identifying rationality with the results of some specific process of reasoning, it is suggested here that rationality is to be identified with the process itself. The emphasis is shifted from some set of justified statements and a privileged way of proving them to an undetermined process of eliminating unreasonable ones. Not any process of reasoning is characteristically rationalist, however, but only processes which serve the aim of critical debate. This enlargement in scope is restricted only by one condition, namely, the imperative to eliminate contradictions. In this way, rationality, as a process of refutation, can and should accept various non-classical logical systems.
The proposed analysis reveals the conclusion, that Reductio ad Absurdum can not lead to consensus. Disagreement and divergence of views is a perpetual state. Rationality changes its nature and becomes basically partial; a never ending process of arguing. It can, however, circumscribe the threat of unreasoned relativism and nihilism. It can place disagreement and divergence of views in the constraints of reason and justifiability.